While we believe that focusing on tips and tricks won’t lead to success on the GMAT, and that mastering core underlying concepts and skills is essential, this doesn’t mean that test taking strategy isn’t critically important.
In fact, while we do believe that building core mathematical and verbal skills is mandatory to do well on the GMAT, we have found that many test prep firms encourage students to spend far too much time learning mathematics. While math concepts are a critical component of the GMAT, it’s simply not a math test. Preparing for the GMAT as if it is a math test is not the most effective or efficient path to a 700 score or better on the exam. To understand what I mean in more detail, let’s start with some common test prep myths.
Common GMAT Myths
Here are four common beliefs about the GMAT that I consider a myth:
- The mathematics concepts covered can be quite advanced
- If you just “aren’t a math person” you’ll really struggle
- English/reading sections are always easier to prepare for
- Doing well on standardized tests is primarily about applying the right tips and tricks
In fact, the mathematics on the GMAT does not typically go beyond grade 9 or 10 in the U.S. education system. There are some topics, like probability or number theory, that violate this rule because many U.S. students learn these particular topics a little later (and sometimes not at all), but the basic idea is that the math itself is somewhat basic. You are just asked to apply the math knowledge creatively and using heavy doses of critical thinking.
Myths 2 and 3 go hand in hand. Students often describe themselves as “not math people.” While it is true that having a mental block around math that causes high amounts of stress can result in a significant reduction of your ability to perform well on the quant section, this is typically almost an emotional or perhaps psychological issue, not necessarily an issue around your cognitive ability to do the math. In many cases, people who were really struggling but who can find a way to relax, listen, and trust a tutor, soon find that the math concepts they need to know are not fundamentally that complicated, and they can begin to comfortably apply them with increasing success.
On the other hand, fundamentally weak verbal or reading skills are much more difficult to overcome. Your ability to understand an argument and notice changes in tone and style of an author can be highly intuitive and the result of years and years of reading and writing. It is very often easier to teach math skills than verbal skills, yet far fewer students seek tutoring because they are not “verbal” people. Over the course of 20 years, a person who enjoys regularly reading and writing, compared to someone who doesn’t enjoy reading and writing, may amass 10 or even 20 times as much “indirect” GMAT verbal “practice.” What is indirect GMAT verbal practice? Well, simply reading the Economist regularly and thinking about the main theme/argument of the article is powerful “indirect” GMAT verbal practice.
Number 4 is a tricky myth. On the one hand, it really is false. The GMAT can’t be overcome through tips and tricks. It is often considered the most difficult of the major standardized tests (ACT, SAT, GRE, LSAT), and requires mastery of mathematics and verbal concepts so that you can creatively apply them to difficult questions. Tips and tricks just won’t be enough. On the other hand, it is fundamentally a test about critical thinking, reasoning, logic, problem solving, and strategy, which can often make it seem like tips and tricks are important. In fact, as in business more generally, the “right” answer is often arrived at by considering multiple sources of information and reasoning through to the best answer. This is often a much different thought process than one might employ in a typical math class, where you are recalling a formula and somewhat robotically applying it.
The best way to understand what I mean is through an example.
The Importance of Strategy and Critical Thinking: An Example from Trigonometry
Observe the image below. A real GMAT question asks, what is the approximate perimeter of the square through which there is a diagonal line of length 20?
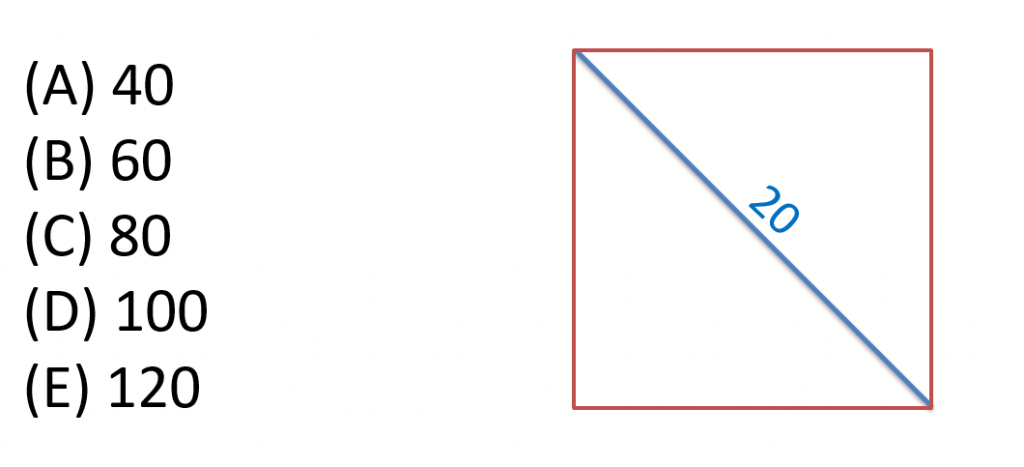
A common, but far less than ideal, way to approach this question is to notice that a diagonal line through a square means that you can apply the Pythagorean theorem, because a right triangle has been created. You can label the sides a and b, and notice that a^2 + b^2 = 20^2, or a^2 + b^2 = 400. Then, you can note a^2 + b^2 is really equal to a^2 + a^2, since we are talking about a square (i.e. all four sides are the same).
So, 2 (a^2) = 400, or a^2 = 200. At this point, you might roughly notice that a is between 14 and 15, and therefore the areas of the square is about 60. But, if you are treating this like a math problem, you probably try to take the square root of 200 at this point, and notice that it’s not whole number. Even if you had a calculator, which would be impossible for you to have on the GMAT, you’d notice that a = 14.142. This means that the perimeter is ~57. B is the right answer, and generally the GMAT is looking for approximate values, so 57 is close enough go 60.
Approaching the problem this way though is not ideal, even though many GMAT prep companies would probably tell you to do just that. You are doing a lot of math, and coming up against the square root of 200 at the end, which is not a whole number and potentially causes you some lost time as you think about whether you’ve gone wrong somewhere. You can still, of course, quickly get to the right answer by noting that something between 14 and 15 is the square root of 200.
Consider the following approach instead:
- Note that if the diagonal is 20, it would be impossible for each side to be 20. You know this from the Pythagorean theorem, but without having to do any calculations. The other sides have to be less than 20.
- So, this means that 80 for the perimeter is too large, and this also means that choices D and E are incorrect.
- Now, you might try A), since that would be the extreme on the low end. 40 means that each side is 10. If you’ve done any amount of practice for the GMAT around trigonometry, and you know what the Pythagorean theorem says, you’ll probably quickly realize that 10^2 + 10^2 = 200, and that doesn’t equal 20^2. 10 is too small and can’t be right.
- So far, you haven’t really done any calculating that isn’t very simple, and you know the answer is B, because you’ve eliminated everything else.
I always find this problem to be a great example of how critical thinking and problem solving is fundamental to GMAT success. Did you need to know a math concept to answer this correctly? Well yes, you had to understand the Pythagorean theorem. But, were you well served by simply turning it into a math problem and working out the answer? No. Instead, you think critically about what is being asked, eliminate obviously incorrect answers, and using the process of elimination, quickly arrive at the right answer with a high degree of confidence, which saves you time.
Many, many GMAT problems can be approached “strategically” like this.
About the Author
Mark Skoskiewicz founded MyGuru, a boutique tutoring and test prep company with a focus on in-person as well as online GMAT tutoring. He holds a B.S. from Indiana University and an MBA from the Kellogg School of Management at Northwestern University.





Leave A Comment